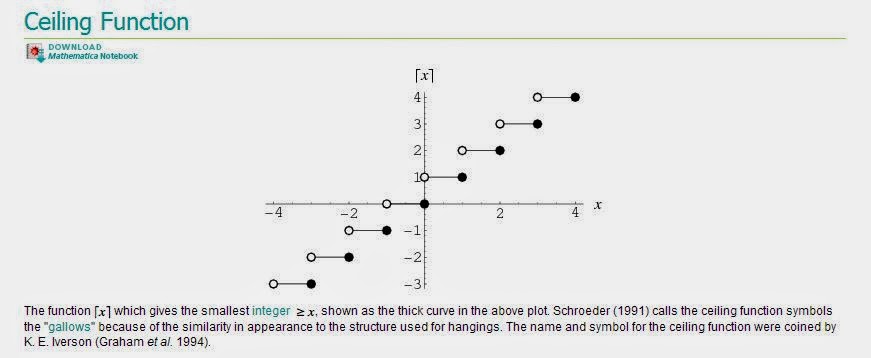
quinta-feira, 7 de agosto de 2014
Python
“When you have eliminated the impossible, whatever remains, however improbable, must be the truth.”
(A. Conan Doyle, The Sign of Four)
(A. Conan Doyle, The Sign of Four)
quarta-feira, 6 de agosto de 2014
domingo, 6 de julho de 2014
sábado, 28 de junho de 2014
quinta-feira, 19 de junho de 2014
domingo, 15 de junho de 2014
sábado, 14 de junho de 2014
domingo, 8 de junho de 2014
18-02-Multivariable-Calculus-fall-2007
Multivariable Calculus
Lagrange multipliers with two variables Mathlet from the d'Arbeloff Interactive Math Project. (Image courtesy of Jean-Michel Claus.)
Instructor(s)
Prof. Denis Auroux
MIT Course Number
18.02
As Taught In
Fall 2007
Level
Undergraduate
Translated Versions
Course Features
Course Description
This course covers vector and multi-variable calculus. It is the second semester in the freshman calculus sequence. Topics include vectors and matrices, partial derivatives, double and triple integrals, and vector calculus in 2 and 3-space.
MIT OpenCourseWare offers another version of 18.02, from the Spring 2006 term. Both versions cover the same material, although they are taught by different faculty and rely on different textbooks. Multivariable Calculus (18.02) is taught during the Fall and Spring terms at MIT, and is a required subject for all MIT undergraduates.
18-02sc-multivariable-calculus-fall-2010
This unit covers the basic concepts and language we will use throughout the course. Just like every other topic we cover, we can view vectors and matrices algebraically and geometrically. It is important that you learn both viewpoints and the relationship between them.
» Session 15: Equations of Lines
» Session 16: Intersection of a Line and a Plane
» Session 17: General Parametric Equations; the Cycloid
» Session 18: Point (Cusp) on Cycloid
» Session 19: Velocity and Acceleration
» Session 20: Velocity and Arc Length
» Session 21: Kepler's Second Law
» Problem Set 3
The Geometry of Euclidean Space
1 The Geometry of Euclidean Space .......................31
2 Differentiation,,,,,,,,,,,,,,,,,,,,,,,,.,,,,,,,,,,,,................124
3 Higher-Order Derivatives; Maxima and Minima...211
4 Vector-Valued Functions ................................. 291
5 Doublr and Triple Integrals ............................... 347
6 The Change of Variables .................................. 398
7 Integrals Over Paths and surfaces ...................... 451
8 The Integrals Theorems of Vector Analysis ....... 548
domingo, 1 de junho de 2014
sábado, 31 de maio de 2014
terça-feira, 27 de maio de 2014
divergência do rotacional, rotacional do gradiente
Vector Calculus Identities
The divergence of the curl is equal to zero:
The curl of the gradient is equal to zero:
| More vector identities |
terça-feira, 20 de maio de 2014
segunda-feira, 19 de maio de 2014
Integrais de linha de campos escalares relativamente ao comprimento de arco.
Integrais de linha no caso geral. Aplicações à Física. Integrais de linha de campos vectoriais.
Campos conservativos campos de gradientes e rotacional. Domínios simplesmente conexos.
Teste para independência de caminho.
Superfícies parametrizadas no espaço euclidiano tridimensional.
Integrais de superfície de funções escalares.
Áreas de superfícies.
Teorema de Green no plano e aplicações.
Integral de um campo de vectores sobre uma superfície. Teorema da Divergência (Gauss) e teorema de Stokes.
.
Integrais de linha no caso geral. Aplicações à Física. Integrais de linha de campos vectoriais.
Campos conservativos campos de gradientes e rotacional. Domínios simplesmente conexos.
Teste para independência de caminho.
Superfícies parametrizadas no espaço euclidiano tridimensional.
Integrais de superfície de funções escalares.
Áreas de superfícies.
Teorema de Green no plano e aplicações.
Integral de um campo de vectores sobre uma superfície. Teorema da Divergência (Gauss) e teorema de Stokes.
.
sábado, 10 de maio de 2014
sexta-feira, 9 de maio de 2014
terça-feira, 6 de maio de 2014
segunda-feira, 5 de maio de 2014
sexta-feira, 2 de maio de 2014
terça-feira, 29 de abril de 2014
segunda-feira, 28 de abril de 2014
Marsden Tromba Vector Calculus
Internet Supplement for Vector Calculus
*
*
1 The Geometry of Euclidean Space ........................ 31
2 Differentiation,,,,,,,,,,,,,,,,,,,,,,,,.,,,,,............... 124
3 Higher-Order Derivatives; Maxima and Minima........ 211
4 Vector-Valued Functions ................................. 291
5 Double and Triple Integrals ............................... 347
6 The Change of Variables .................................. 398
7 Integrals Over Paths and surfaces ....................... 451
8 The Integral Theorems of Vector Analysis ............. 548
2 Differentiation,,,,,,,,,,,,,,,,,,,,,,,,.,,,,,............... 124
3 Higher-Order Derivatives; Maxima and Minima........ 211
4 Vector-Valued Functions ................................. 291
5 Double and Triple Integrals ............................... 347
6 The Change of Variables .................................. 398
7 Integrals Over Paths and surfaces ....................... 451
8 The Integral Theorems of Vector Analysis ............. 548
Subscrever:
Comentários (Atom)